). It should be clear that $x_4 = 0$ and that $x_1 = a$, $x_2 = b$ and $x_3 = c = \frac {-a-2b} {3}$. An online nullspace calculator can find a basis for the null space of the matrix by following these steps: The null space always contains a zero vector, but other vectors can also exist. The proof will proceed as follows. Consider the following example. Learn more about Stack Overflow the company, and our products. Therefore \(m=n\). However, since $V$ is itself a vector space, most of the axioms are basically satisfied already. Now, suppose that $\alpha \in \Bbb R$. barbecue festival 2022; olivia clare friedman net worth. Thus $S$ is closed under addition. Using this online calculator, you will receive a detailed step-by-step Find the dimension of \(\mathbb{P}_2\). In particular, \(\left [ \begin{array}{rr} 0 & 0 \\ 0 & 1 \end{array} \right ]\) is not in \(\mathrm{span} R\). WebTo calculate projection onto one-dimensional subspace space, you can simply take unit vector u generating this subspace and then and Clarify mathematic problem If you want to improve your theoretical performance, you need to put in the work. We take r (until it is zero) and use it to delete the entries below it. Why is it forbidden to open hands with fewer than 8 high card points? Notice that the dimension is well defined by Corollary \(\PageIndex{2}\). Then \(V\) is a subspace of itself. WebTo prove a subset is a subspace of a vector space we have to prove that the same operations (closed under vector addition and closed under What do our customers say? A subspace is just a vector space 'contained' in another vector space. A proper subset contains few elements of the original set but an improper subset contains each element of the Original set, as well as an empty set and subset calculator, which gives the number of the proper and improper subset in a set. Then corresponding to the above vectors in \(V\) we would have the following vectors in \(\mathbb{R}^{4}.\) \[\left [ \begin{array}{c} 1 \\ 1 \\ 2 \\ 0 \end{array} \right ] ,\left [ \begin{array}{c} 2 \\ 2 \\ 4 \\ 1 \end{array} \right ] ,\left [ \begin{array}{c} 1 \\ 2 \\ 2 \\ 2 \end{array} \right ] ,\left [ \begin{array}{r} 2 \\ -3 \\ 4 \\ 1 \end{array} \right ] ,\left [ \begin{array}{c} 1 \\ 2 \\ 3 \\ 1 \end{array} \right ]\nonumber \] Now if we obtain a subset of these which has the same span but which is linearly independent, then the corresponding vectors from \(V\) will also be linearly independent.
, where
From the source of Lumen Learning: Using Matrices to Solve Systems of Equations, Matrix Equations, Writing a System of Equations with Matrices, Matrices and Row Operations, Elementary Row Operations (ERO), Produce Equivalent Matrices Using Elementary Row Operations. Share Cite Follow edited Mar 27, 2013 at 0:07 answered Mar 27, 2013 at 0:01 amWhy 1 Nice hints! It is important to note that a basis for a vector space is not unique.
Site design / logo 2023 Stack Exchange Inc; user contributions licensed under CC BY-SA. The idea is used to destroy as many matrix elements as possible. In the established symbols, we write || = 0. It has a dimension of 0 and contains only a zero vector. Webinterior angle sum regular million-gon. When trying to determine the nullity and kernel of a matrix, the most important tool is Gauss-Jordan Elimination. Why is it forbidden to open hands with fewer than 8 high card points? Let \(r(x)\) represent this sum. For example, your $U_1$ is a set of matrices, not simply a matrix. Let \(V\) be a finite dimensional vector space and let \(W\) be a non-zero subspace. Find a matrix in row linear-independent. It only takes a minute to sign up. 
WebDimension of a subspace calculator Let be a real vector space (e. Our Target is to find the basis and dimension of W. The Column Space Calculator will find a basis for the column space of a Clarify math problem. components of these vectors may be real or complex numbers, as well as parametric expressions. Let \(p(x), q(x)\) be polynomials in \(W\). z z z z . Recall Example 9.3.4 in which we added a matrix to a linearly independent set to create a larger linearly independent set. 
basis
Browse other questions tagged, Start here for a quick overview of the site, Detailed answers to any questions you might have, Discuss the workings and policies of this site. As you can see, we can verify the two points easily in a general case. Let \(ax^2 + bx + c\) be an arbitrary polynomial in \(\mathbb{P}_2\). For any vectors \(\vec{w}_1, \vec{w}_2\) in \(W\), \(\vec{w}_1 + \vec{w}_2\) is also in \(W\). system of vectors. Then there exists \(\left\{ \vec{u}_{1},\cdots ,\vec{u}_{k}\right\} \subseteq \left\{ \vec{w}_{1},\cdots ,\vec{w}_{m}\right\}\) such that \(span \left\{ \vec{u}_{1},\cdots ,\vec{u}_{k}\right\} =W.\) If \[\sum_{i=1}^{k}c_{i}\vec{w}_{i}=\vec{0}\nonumber \] and not all of the \(c_{i}=0,\) then you could pick \(c_{j}\neq 0\), divide by it and solve for \(\vec{u}_{j}\) in terms of the others. The reduced row echelon form of the matrix: To find the null space, solve the matrix equation: [1 0 0 0 0 1 0 0 0 0 1 0 0 0 0 1] [x_1x_2x_3x_4] = [0 0 0 0]. 
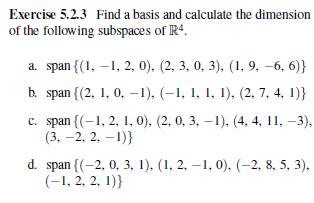Write the vector v = (2, 2, 2) as the sum of a vector in S and a vector orthogonal to S. Let \(\vec{w}_1, \vec{w}_2\) be in \(\left\{ \vec{0} \right\}\). 1 Answer. so $(\alpha x_1, \alpha y_1, \alpha z_1) = \alpha(x_1,y_1,z_1) \in S$. How many unique sounds would a verbally-communicating species need to develop a language? If the matrix has no columns without initials, then the null space is trivial. of the vectors
Here, we will discuss these concepts in terms of abstract vector spaces. The next theorem is an essential result in linear algebra and is called the exchange theorem. Let \(W \subseteq \mathbb{P}_2\) be all polynomials of degree two or less which have \(1\) as a root. It is assumed here that \(n<\infty\) and therefore such a vector space is said to be finite dimensional. Consider the following example of this concept. compare electrolytes in sports drinks science project. How to prove the zero vector is a subspace of upper triangle matrices? Multiply a string by a non-zero constant; Enter the size of rows and columns of a matrix and substitute the given values in all fields. I've been working through some exercise sheets for uni and for the life of me I can't work out how to do the following question: For each of the following, either use the subspace test to show that the given subset, Since \(V\) satisfies the vector space axioms it also satisfies the three steps of the subspace test. b. WebThe Null Space Calculator will find a basis for the null space of a matrix for you, and show all steps in the process along the way. Next, the null space of matrix calculator does similar to the middle row. When determining spanning sets the following theorem proves useful. If there are exist the numbers
It follows that \(p(1) = 0\) and \(q(1) = 0\). You have the desired basis. P is a subset of Q, namely PQ, and P is not equal to Q, that is, PQ. linearly independent vectors. If set P has {A, B} and set Q has {A, B, C}, then P is a subset of Q because there are also elements of set P in set Q. Browse other questions tagged, Start here for a quick overview of the site, Detailed answers to any questions you might have, Discuss the workings and policies of this site. The rank-nullity theorem is defined as, Nullity X + Rank X = the total number of attributes of X (that are the total number of columns in X). Everybody needs a calculator at some point, get the ease of calculating anything from the source of calculator-online.net. However, an onlineDeterminant Calculator allows you to calculate the determinant of the given matrix input elements. is called
Why exactly is discrimination (between foreigners) by citizenship considered normal? The number of proper subsets of a given sub-set is \(2^n-1\). What is the connection between linear algebra and geometry? Therefore at least one \(c_i\) is nonzero. So, the basis is just the combination of all linearly independent vectors. For further assistance, please Contact Us. Then it would follow that \(\vec{x}_{1}=\vec{0}\) and so \(\left\{ \vec{x} _{1},\cdots ,\vec{x}_{r}\right\}\) would not be linearly independent. Suppose \(n > m.\) Then since the vectors \(\left\{ \vec{u} _{1},\cdots ,\vec{u}_{m}\right\}\) span \(V,\) there exist scalars \(c_{ij}\) such that \[\sum_{i=1}^{m}c_{ij}\vec{u}_{i}=\vec{v}_{j}.\nonumber \] Therefore, \[\sum_{j=1}^{n}d_{j}\vec{v}_{j}=\vec{0} \text{ if and only if }\sum_{j=1}^{n}\sum_{i=1}^{m}c_{ij}d_{j}\vec{u}_{i}= \vec{0}\nonumber \] if and only if \[\sum_{i=1}^{m}\left( \sum_{j=1}^{n}c_{ij}d_{j}\right) \vec{u}_{i}=\vec{ 0}\nonumber \] Now since \(\{\vec{u}_{1},\cdots ,\vec{u}_{n}\}\) is independent, this happens if and only if \[\sum_{j=1}^{n}c_{ij}d_{j}=0,\;i=1,2,\cdots ,m.\nonumber \] However, this is a system of \(m\) equations in \(n\) variables, \(d_{1},\cdots ,d_{n}\) and \(m
Ouellet Baseboard Heaters,
James Preston Kennedy Louisiana,
Articles S